5 - The Spin Quantum Number
Abstract (TL;DR):
The Spin Quantum Number begins with an experiment by Otto Stern and Walther Gerlach, which demonstrated that a beam of atoms split evenly into two. This is due to a property of the electrons that causes them to generate their own magnetic field and, consequently, their own internal momentum. This momentum causes them to behave in two, specific ways, called “spins”.
—
Originally posted by geometricanimations
This is it! We have finally reached the conclusion of orbitals. I’m sure it felt like the Frieza Saga, but condensing atomic orbital theory into only a few articles isn’t half bad. Of course, I’ll leave it to you to tell me how I can improve to make this a better story for you. Because, like electrons, we all have to work together here.
In any event, let’s march on and be done with these orbitals. Ah…I can already hear the cheers from the Chemistry 101 students reading this.
Be sure to check out Part 4 for this one. You can’t understand where this one begins without seeing where the other one ends.
Now, it is time to explain the last quantum number.
The Quantum Dive - Internal Energy
Stern-Gerlach Experiment
Electrons are trying to get rid of their energy in any way possible. Orienting themselves in a way that lowers energy is a good starting point, but they still have plenty of internal energy. In fact, electrons have their own, internal angular momentum, separate from the orbital angular momentum (l).
The Stern-Gerlach Experiment Setup
(1) The emitter for the silver atom beam. (2) The silver atom beam. (3) The nonuniform magnets (north and south poles are different shapes, distorting the magnetic field). (4) What they expected the result to be. (5) What the result actually was.
This was determined after a 1922 experiment by German physicists Otto Stern and Walther Gerlach. The aptly named Stern-Gerlach Experiment involved firing a beam of silver atoms* through a nonuniform, magnetic field and observing the results on a detecting wall at the end. The result, surprisingly, was that the beam was deflected in only two directions, landing flush against a plate in two spots.
But how could that be? There’s no way that there should be two, specific places in which the atoms ended up.
If you think about it, if you threw twenty bar magnets at a magnetic wall past two huge magnets and looked at where they ended up, they would all be stuck on the wall in specific places according to their poles. After all, you have no idea what direction those magnets were when you threw them or how much attraction or repulsion one magnet had than another one, so you should have no idea exactly how they will come out. Shouldn’t it have been the same for these atoms?
In order to explain the significance of this inconsistency, I’ll need to diverge for a second into the physical quantity of momentum.
Types of Momentum
Given that we know, from classical linear momentum calculation that there must a velocity, some speed over some time from some position which is usually produced by some force (say that ten times fast), and a mass, or the amount of matter an object has. This tells you how much, in numerical form, an object moves. In fact, you likely already knew the definition of “momentum” without knowing how to put it in words. Classical angular momentum is almost completely the same. The only difference is that an axis is involved, around which your mass rotates.
Thus, the two relative quantities are the moment of inertia and angular velocity. The latter is simply the rate at which rotation occurs. On the other hand, the moment of inertia, also known as the angular mass, is used to depict how much torque, or rotational force, you need to move an object a certain distance around an axis with respect to a certain position. The classic example of this is the tightrope walker.
If it was super easy to cause the one walking on a tightrope to flip around the rope (if gravity didn’t exist), then you would say that their moment of inertia is low. But when one walks across a rope you usually see them stick their arms out or hold a long rod. That single act increases the moment of inertia because you have changed the center of the mass, where the force acts. That is, more rotational force would be needed to rotate the walker.
Now, Matthew, why did you go through the effort of explaining momentum here? Patience, reader! The science will wrap together in a nice ribbon. Just in time for Christmas.
Magnetism
By the way, if you orient your right-hand’s four fingers so that they are going along the path of the electron and then point out your thumb, that gives you the direction of the magnetic field. Every time. It’s called the Right-Hand Rule.
Image via SchoolPhysics
I mentioned, from the now legendary Part 2, that electrons, as charged particles, can generate a magnetic field just by moving. It so happens that we know that electrons are “orbiting” the nucleus, and therefore, they must be exhibiting some sort of magnetic field as it is repeatedly revolving in this closed loop. But we never talked about what that field is doing. It wouldn’t make sense for the magnetic field to just disregard the electron and there is definitely no reason for the electron to not feel the force of the magnetic field.
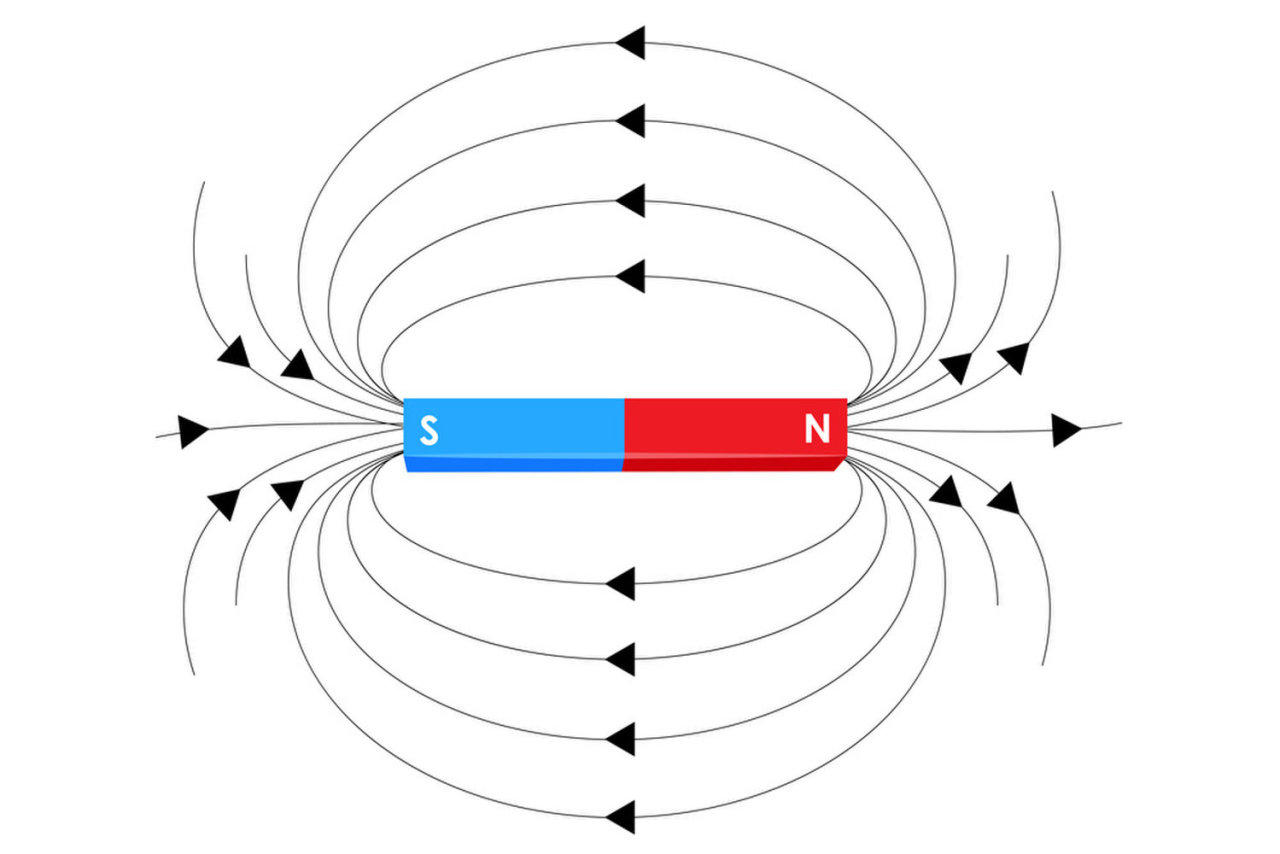
What would happen if you were to hold your hand on a ball lying on a table and then push your hand forward? The ball would roll in the direction your hand moves. It’s the same with electrons – they experience a rotational force, torque, from the magnetic field. The rotating electron creates another effect, according to electrodynamics. It now becomes a natural magnetic dipole, which is an object that generates magnetic fields from two opposite poles (like the north and south pole of the Earth). In many ways, their magnetic effects are just tiny bar magnets. Electrons, too, have a “north” and “south” pole, or, more specifically, two poles at which their magnetic fields are strongest.
So, let’s do a roundup of what we just learned.
Your common bar magnet is a dipole. The Earth is also one.
Image via Live Science
Electrons generate magnetic fields which creates torque on them causing them to rotate and become similar to bar magnets.
This is where the concept of an electron’s internal angular momentum comes from. Indeed, electrons exhibit their own magnetic force from their rotation, and, coupled with their motion, create their own internal angular momentum!
This is the origin of the theoretical “spin” that electrons have. Although we, realistically, don’t know if the electrons are spinning or not, this is just a name we give conventionally. You will learn why our convention fails us, especially when it comes to quantum mechanics, later.
With all of that said…how did the electrons only end up in two places in the Stern-Gerlach Experiment?
Spin Quantum Number (s)
Stern and Gerlach were smart men. They knew, from Bohr’s prior experimentation of the electron, that it must be quantized. In other words, the electron had to exist in specific, discrete states. So when they crafted this experiment, they expected a quantized result. That is exactly what they saw - atoms being redirected by the magnets to two, specific areas.
But just because they were correct in their hypothesis doesn’t mean their foundation was solid – a lesson for all of you upcoming scientists.
Although that’s a result that you could hypothesize based on an expectation gained after seeing the ice in a glass of water melt, the foundation is all wrong.
Image by Bill Watterson
The quantum mechanical theory of the time wasn’t correct. Their conclusion that the result was due to the quantization of the electron was not the reason for the quantized result. The result was due to the electron’s “spin”.
In the presence of an external magnetic field (B), like the one Stern and Gerlach fired silver atoms through, electrons have two half-spins.
Remember, we don’t believe the electrons actually spin. This is just a representation of the quantum mechanical concept.
Image by William Reusch via Michigan State University
Remember, the electrons became magnetic dipoles, meaning, according to how their magnetic field influenced them, they would act like a “north pole” or a “south pole”. What happens when you push the north pole of a bar magnet into the north pole of another magnet? They repel each other! What do you think happens when you push an electron with a north pole “spin” into a north pole magnet? Yes, they repel each other. That goes for an electron with a south pole “spin” and a south pole magnet as well.
It was because of the electron’s angular momentum was directed in two, specific directions that the result of the Stern-Gerlach experiment showed registered silver atoms at two, specific spots on the plate. We call each of the electrons two rotations “half-spins”, since one beam was split evenly into two.
The Higgs Boson
All elementary particles that hold these spins equal to ½ are called fermions, whereas if their spin value was 1 (what the value would be if the one beam went straight through without any division), it would be called a boson, a word you’ve likely heard before if you’re into physics. These are the two categories in which all particles in the universe reside.
- Image of the Higgs Boson, also known as the God Particle
Summary of The FourQuantum Numbers
So…how about another summary?
The Principle Quantum Number (n) determines the energy level, and thus, the electron’s shell. It is conventionally shown with a number from 1 to 5. The Orbital Quantum Number (l) determines where the electrons are with respect to the nucleus. It shows the sub-shell and is typically denoted with a letter (s, p, d or f) according to its number (0, 1, 2 and 3). The Magnetic Quantum Number (m) tells how many subshells there are. It is determined by looking at the subshell and taking the range from -l to l. Lastly, the complex Spin Quantum Number (s) determines whether the electron’s “spin” is +½ or -½.
Lastly, there’s the Pauli Exclusion Principle, watching over these Quantum Numbers to make sure they behave themselves. No two electrons can have the same quantum mechanical state within the same atom. It was the aforementioned spin quantum number that proved this; remember that the electrons in the Stern-Gerlach did not simply mix – there was a distinction between +½ and -½. Furthermore, given that the Principle only works with differing quantum mechanical states, only fermions follow this Principle.
Image via futurespaceprogram
This opens up a very important piece of orbital theory.
That spin quantum number shows a great deal, indeed, folks. Because that Pauli Exclusion Principle makes it so that each subshell can only have two electrons – one with the positive spin and one with the negative spin.
And that, my friends, will lead us into the Aufbau Principle, the ending of our journey…Which we will discuss next time.
Please ask questions if you are lost somewhere in these five parts. And share amongst all of your friends – not just the ones interested in science. We can all learn. We just need to light the fire.
* If you understood everything up to this point, you might be able to reason why silver atoms were used. I want to ask you why and give you these three hints to help you:
Use the periodic table.
Orbital Quantum Number
Electrons and Protons